Implementation of Subthreshold Adiabatic Logic for Ultralow Power Application

Source : VHDL & Verilog HDL ( DSCH3/MICROWIND)

Abstract:

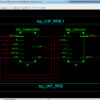
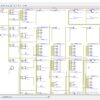
The Subthreshold adiabatic logic for Ultralow power application is a novel approach is efficient in low speed operations, where power consumption and longevity are the pivotal concerns instead of performance. Here, we are implementing the adiabatic logic gates and implementing CLA 8-bit, it will compared to the normal logic gates, the adiabatic logic makes a more power consumption and also increasing speed. The schematic and layout of a 4-bit carry look ahead adder (CLA) has been implemented to show the workability of the proposed logic. The effect of temperature and process parameter variations on sub threshold adiabatic logic-based 4-bit CLA has also been addressed separately. Post layout simulations show that sub threshold adiabatic units can save significant energy compared with a logically equivalent static CMOS implementation.

List of the following  materials will be included with the Downloaded Backup: